Alexandru Zaharescu
 The Circle Problem, the Divisor Problem, and Bessel Function Series
The Circle Problem, the Divisor Problem, and Bessel Function Series
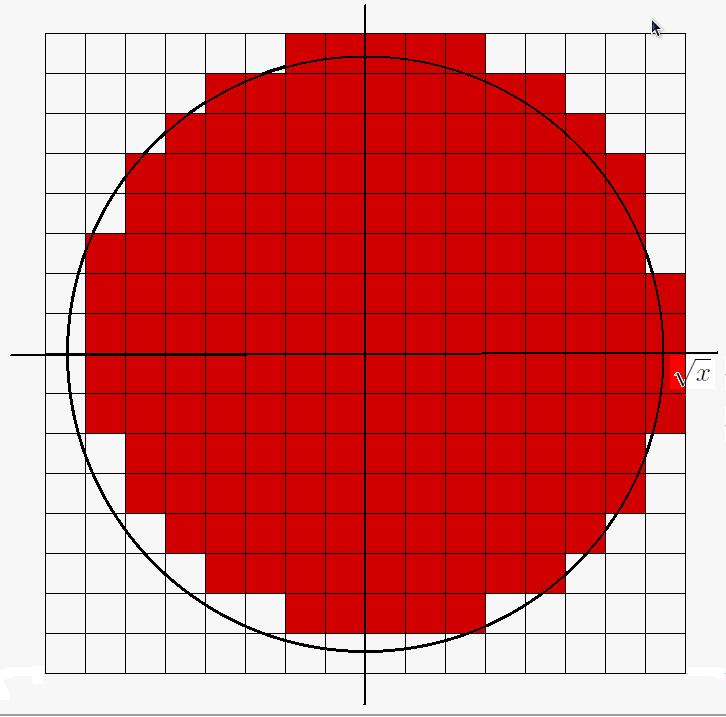
The circle problem and the Dirichlet divisor problem are two of the most difficult, long-outstanding problems in analytic number theory. The problems pertain to obtaining accurate asymptotic formulas for certain sums that involve the number of representations of an integer number n as a sum of two squares and, respectively, the number of divisors of the integer n. Both problems have simple geometric interpretations, involving the number of points with integer coordinates inside a large circle, and, respectively, the number of points with positive integer coordinates that lie under a certain hyperbola. Many mathematicians have studied these problems, and some partial results have been obtained.
The most successful approaches so far are based on two formulas by Hardy and Voronoi that, respectively, connect the circle problem and the divisor problem to certain series of Bessel functions. Ramanujan discovered two formulas that are related to but significantly more complicated than the formulas provided by Hardy and Voronoi.
Until recently, no proof was known for either of Ramanujan’s formulas. In a series of joint papers with Bruce Berndt and Sun Kim, Professor Zaharescu established by different methods various results related to Ramanujan’s formulas. Recently, the group succeeded in proving Ramanujan’s first formula. During his Center appointment, Professor Zaharescu will work toward proving the second, more difficult formula of Ramanujan.
